Mathematics 101
- Reminder of Fundamentals
- Advanced Arithmetics
- Introduction to Geometry
- In-depth Geometry
- Deeper into Numbers
- Further into Algebra
- Elementary Statistics & Probability
- Advanced Statistics, Probability
- Mathematical Logic
- Calculus
- Calculus
- Trigonometry I
- Trigonometry II & Conclusion
Advanced Arithmetics
Understanding Fractions and Decimals
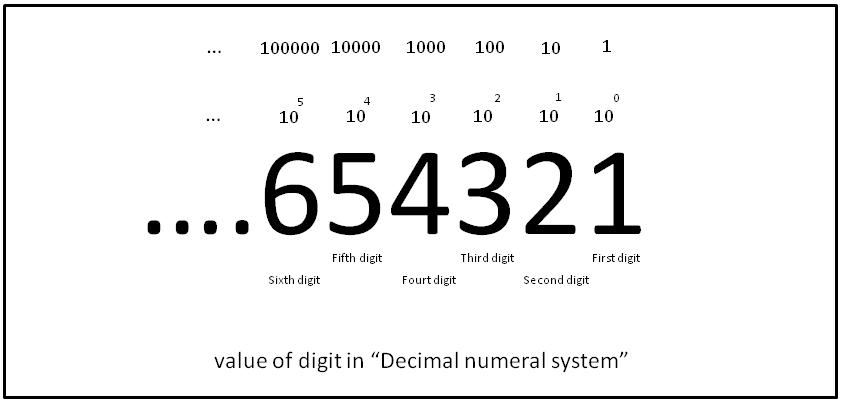
Numeral system with ten as its base.
Introduction
Fractions and decimals are fundamental concepts in mathematics that are used in a variety of real-world applications. They are used to represent quantities that are not whole numbers and are essential for understanding more advanced mathematical concepts.
Understanding Fractions
A fraction is a way of expressing a quantity that is not a whole number. It consists of two parts: the numerator, which is the number above the line, and the denominator, which is the number below the line. The numerator represents the number of parts we have, while the denominator represents the total number of equal parts.
For example, in the fraction 3/4, 3 is the numerator and 4 is the denominator. This fraction represents the fact that we have 3 out of 4 equal parts.
Simplifying Fractions
Fractions can often be simplified by finding a number that both the numerator and the denominator can be divided by. For example, the fraction 6/8 can be simplified to 3/4 by dividing both the numerator and the denominator by 2.
Operations with Fractions
Adding, subtracting, multiplying, and dividing fractions involves a few more steps than the same operations with whole numbers.
- Addition and Subtraction: To add or subtract fractions, they must have the same denominator. If they do not, you must first find a common denominator.
- Multiplication: To multiply fractions, multiply the numerators together and the denominators together.
- Division: To divide fractions, multiply the first fraction by the reciprocal of the second fraction.
Understanding Decimals
Decimals are another way of representing quantities that are not whole numbers. They are based on the concept of tenths, hundredths, thousandths, and so on.
For example, the decimal 0.75 represents 75 hundredths, or 7 tenths and 5 hundredths. This is the same quantity as the fraction 3/4.
Conversion between Fractions and Decimals
Fractions can be converted to decimals, and vice versa. To convert a fraction to a decimal, divide the numerator by the denominator. To convert a decimal to a fraction, determine what place value the decimal is in (tenths, hundredths, etc.) and use that as the denominator.
Rounding Decimals
Rounding decimals involves determining which whole number or decimal place a particular decimal is closest to. This is often used in real-world situations where an exact number is not necessary or practical.
Conclusion
Understanding fractions and decimals is crucial for many areas of mathematics and everyday life. From measuring ingredients for a recipe to dividing a pizza among friends, these concepts are used frequently. By mastering fractions and decimals, you will have a solid foundation for understanding more complex mathematical concepts.