Understanding the Action Principle in Quantum Mechanics

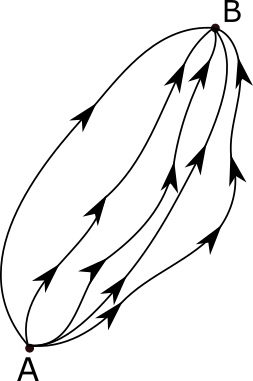
Formal sum or integral over all histories of a quantum system.

The action principle, also known as the principle of least action, is a cornerstone of theoretical physics. It is a principle that states that the path taken by a physical system is such that the action is minimized. This principle is a key component in the path integral formulation of quantum mechanics, which was developed by Richard Feynman.

Introduction to the Principle of Least Action

The principle of least action is a variational principle. According to this principle, the path that a physical system will take between two states is the one for which the action is minimized. The action is a quantity that is calculated by integrating the Lagrangian over time.

The Role of the Action Principle in the Path Integral Formulation

In the path integral formulation of quantum mechanics, the action principle plays a crucial role. The path integral formulation sums over all possible histories of a system, and each history contributes to the sum with a weight that is determined by the action. The histories with the least action contribute the most to the sum.

The Lagrangian and Hamiltonian in the Action Principle

The Lagrangian and Hamiltonian are two quantities that are central to the action principle. The Lagrangian is a function that describes the dynamics of a system. It is defined as the difference between the kinetic and potential energy of the system. The action is calculated by integrating the Lagrangian over time.

The Hamiltonian, on the other hand, is the total energy of the system. It is defined as the sum of the kinetic and potential energy. The Hamiltonian plays a crucial role in the Schrödinger equation, which is the fundamental equation of quantum mechanics.

The Euler-Lagrange Equation and Its Role in the Action Principle

The Euler-Lagrange equation is a differential equation that is derived from the action principle. It provides the equations of motion for a system. The Euler-Lagrange equation is obtained by setting the variation of the action to zero. This equation is used to find the path that minimizes the action, which is the path that the system will take according to the action principle.

In conclusion, the action principle is a fundamental concept in theoretical physics and quantum mechanics. It provides a powerful tool for understanding the dynamics of physical systems.