Superconductivity
- Introduction to Superconductivity
- Theoretical Foundations
- Types of Superconductors
- Superconducting Materials
- Superconducting Phenomena
- Superconducting Devices
- Superconductivity and Quantum Computing
- Challenges in Superconductivity
- Future of Superconductivity
- Case Study: Superconductivity in Energy Sector
- Case Study: Superconductivity in Medical Field
- Case Study: Superconductivity in Transportation
Theoretical Foundations
Ginzburg-Landau Theory: A Deep Dive into Superconductivity
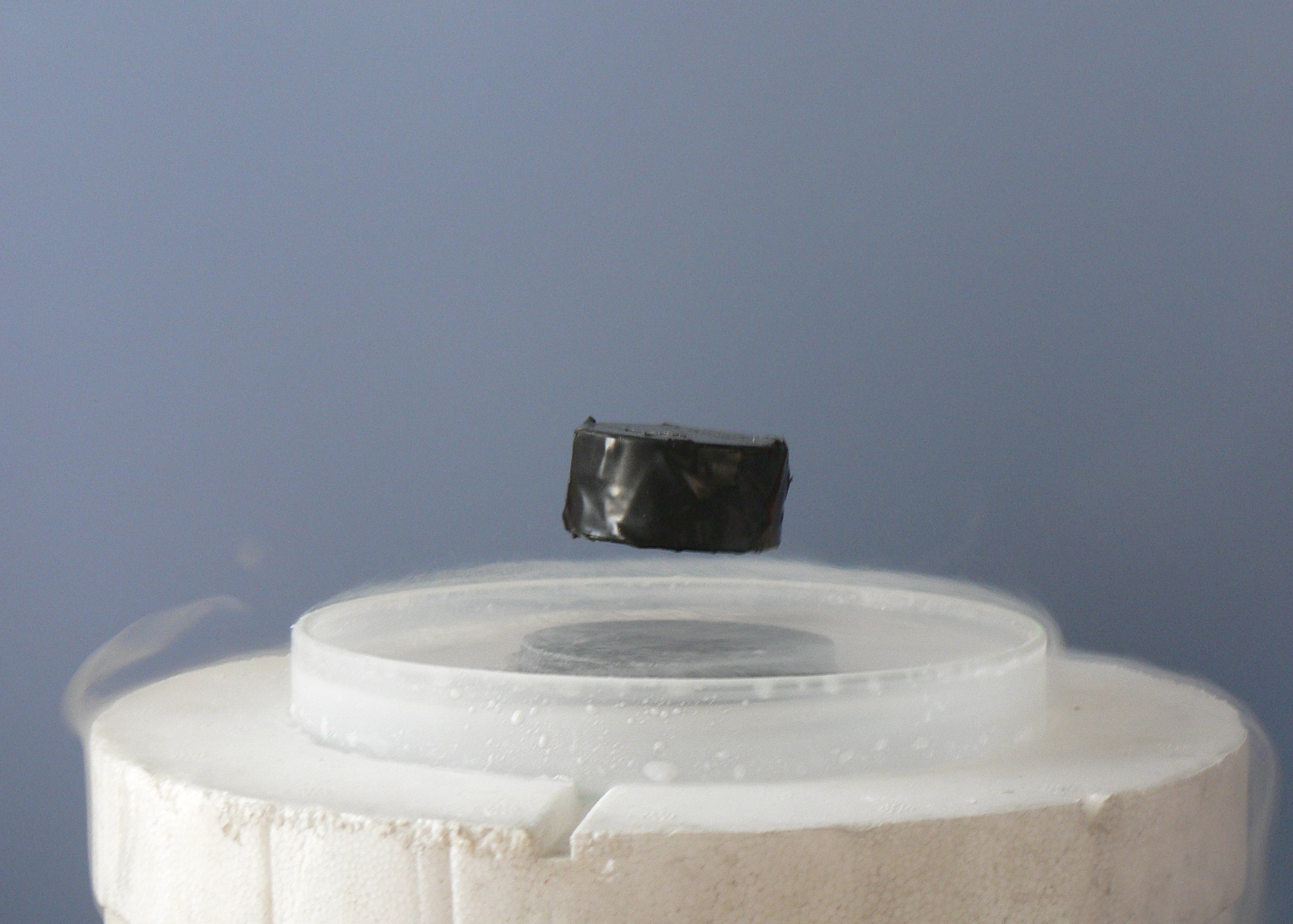
Electrical conductivity with exactly zero resistance.
The Ginzburg-Landau (GL) theory is a phenomenological theory that provides a macroscopic description of superconductivity. Developed by Vitaly Ginzburg and Lev Landau in the 1950s, this theory has been instrumental in our understanding of superconducting materials and phenomena.
Introduction to Ginzburg-Landau Theory
The GL theory was developed to describe superconductivity near the critical temperature, where the BCS theory is not applicable. It is a macroscopic theory, meaning it describes the behavior of a large number of particles, rather than individual particles. The GL theory is based on the concept of an order parameter, a quantity that characterizes the phase of a system.
Order Parameter and its Role in Superconductivity
In the context of superconductivity, the order parameter is a complex number that describes the superconducting state of a material. It is zero in the normal state and non-zero in the superconducting state. The magnitude of the order parameter represents the density of Cooper pairs, and its phase represents the quantum mechanical phase of the Cooper pairs.
The GL theory postulates that the free energy of a superconductor can be expressed as a function of the order parameter. This function has a minimum at the normal state and another minimum at the superconducting state. The transition between these two states occurs when the system's free energy is lowered by changing the order parameter from zero to a non-zero value.
Ginzburg-Landau Equations and their Solutions
The GL theory leads to two partial differential equations, known as the Ginzburg-Landau equations. These equations describe how the order parameter varies in space and time. They can be solved to predict various properties of superconductors, such as the critical magnetic field and the penetration depth of magnetic fields.
The solutions to the GL equations reveal that the order parameter can vary in space, leading to the concept of vortices in superconductors. These vortices, where the order parameter is zero and the magnetic field penetrates the superconductor, are a key feature of type-II superconductors.
Comparison between BCS and Ginzburg-Landau Theories
While both the BCS and GL theories have been successful in describing superconductivity, they have different scopes and limitations. The BCS theory provides a microscopic description of superconductivity, explaining how individual electrons form Cooper pairs. On the other hand, the GL theory provides a macroscopic description, explaining how the superconducting state emerges from the collective behavior of many Cooper pairs.
In conclusion, the Ginzburg-Landau theory has played a crucial role in our understanding of superconductivity. By introducing the concept of an order parameter and deriving the GL equations, this theory has provided a framework for describing and predicting the behavior of superconductors.