Understanding Flux Quantization in Superconductors

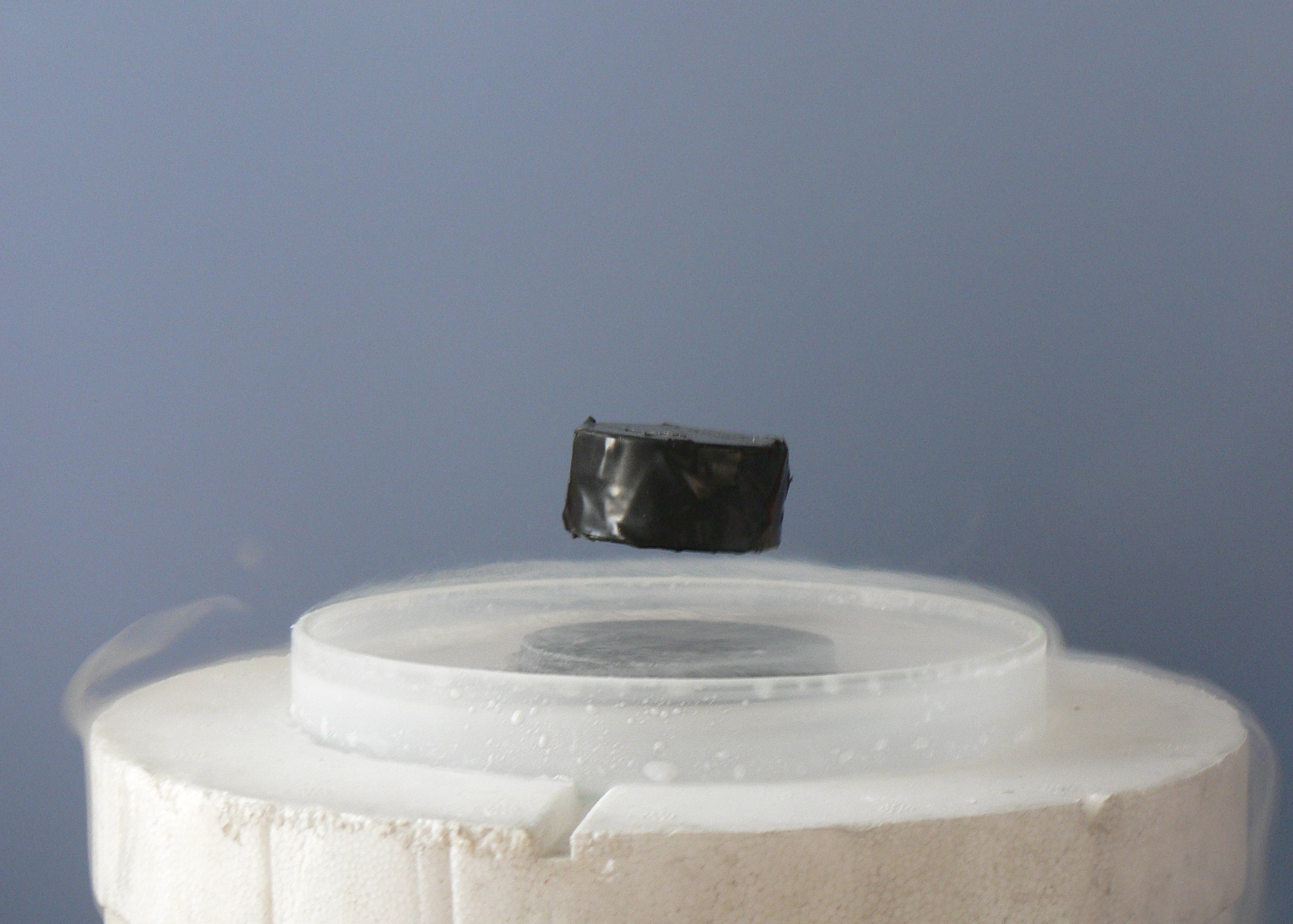
Electrical conductivity with exactly zero resistance.

Flux quantization is one of the most fascinating and important phenomena in superconductivity. It is a direct consequence of the quantum mechanical nature of superconductors and has significant implications for their behavior and applications.

What is Flux Quantization?

In a superconducting loop, the magnetic flux threading the loop is quantized, meaning it can only take on certain discrete values. This is a direct result of the wave-like nature of the superconducting electrons, which form a quantum mechanical state known as a Cooper pair. The quantum mechanical phase of this state must be the same everywhere in the loop, leading to the quantization of the magnetic flux.

The London Equations and Flux Quantization

The London equations, formulated by brothers Fritz and Heinz London in 1935, are a set of phenomenological equations that describe the electromagnetic properties of superconductors. They predict that the magnetic field inside a superconductor is zero, a phenomenon known as the Meissner effect.

However, the London equations also lead to a more subtle prediction: in a superconducting loop, the magnetic flux is quantized. This prediction was confirmed experimentally, providing strong evidence for the quantum mechanical nature of superconductivity.

Experimental Verification of Flux Quantization

The first experimental evidence for flux quantization came from experiments performed by Doll and Näbauer in 1961. They observed that the magnetic flux through a superconducting ring was quantized, with the flux quantum given by the ratio of Planck's constant to twice the electron charge.

This result was a major breakthrough, providing direct evidence for the quantum mechanical nature of superconductivity and the existence of Cooper pairs.

Implications and Applications of Flux Quantization

Flux quantization has significant implications for the behavior and applications of superconductors. For example, it leads to the existence of vortices in type-II superconductors, which play a crucial role in their behavior in high magnetic fields.

Flux quantization is also the basis for the operation of SQUIDs (Superconducting Quantum Interference Devices), which are extremely sensitive magnetometers used in a variety of scientific and technological applications.

In conclusion, flux quantization is a key aspect of superconductivity, providing important insights into the quantum mechanical nature of superconductors and enabling a range of applications. Understanding this phenomenon is therefore crucial for anyone studying or working with superconductors.